Logistisches Wachstum - beschränktes Wachstum: Unterschied zwischen den Versionen
(Die Seite wurde neu angelegt: „ Zurück zum Lernpfad“) |
|||
| Zeile 1: | Zeile 1: | ||
| + | Als <b>logistische Gleichung</b> wird eine Differenzengleichung der Form <math>x_{n+1}=r\cdot x_{n}\cdot(1-x_{n})</math> bezeichnet. Sie wird verwendet, um ein Wachstum mit Schranke zu modellieren. Die <math>\,x_{i}</math> liegen im Intervall zwischen <math>\,0</math> und <math>\,1</math> und beschreiben die Größe einer Population prozentuell. In der Konstanten <math>r</math> sind Parameter wie Wachstumsrate, Sterblichkeit und Ähnliches zusammengefasst. Die zugehörige Differentialgleichung (DGLG) sieht folgendermaßen aus: <br><br> <math>f'(t)=r\cdot f(t)\cdot(1-f(t))</math> | ||
| + | Diese DGLG kann analytisch gelöst werden, zur Vereinfachung wird statt <math>\,f(t) </math> <math>\,f</math>geschrieben: <br><br> | ||
| + | |||
| + | <math> | ||
| + | \frac{df}{dt}=r\cdot f\cdot (1-f) | ||
| + | </math> | ||
| + | |||
| + | <math> | ||
| + | \frac{df}{f\cdot (1-f)}=r \cdot dt | ||
| + | </math> | ||
| + | |||
| + | <math> | ||
| + | \int \frac{df}{f\cdot (1-f)}=\int r \cdot dt | ||
| + | </math> | ||
| + | |||
| + | <math> | ||
| + | \int \frac{A}{f}+\frac{B}{1-f} \cdot df=\int r \cdot dt | ||
| + | </math> | ||
| + | |||
| + | Mittels Partialbruchzerlegung ermittelt man für <math>\,A </math> und <math>\,B </math> jeweils den Wert <math>\,1 </math>. | ||
| + | |||
| + | <math> | ||
| + | \int \frac{1}{f}+\frac{1}{1-f} \cdot df=\int r \cdot dt | ||
| + | </math> | ||
| + | |||
| + | <math> | ||
| + | ln f - ln(1-f) = r\cdot t + C | ||
| + | </math> | ||
| + | |||
| + | <math> | ||
| + | ln \frac{f}{1-f} = r\cdot t + C | ||
| + | </math> | ||
| + | |||
| + | <math> | ||
| + | \frac{f}{1-f} = e^{r\cdot t + C} | ||
| + | </math> | ||
| + | |||
| + | <math> | ||
| + | f = e^{r\cdot t + C}(1-f)=e^{r\cdot t + C}-e^{r\cdot t + C}\cdot f | ||
| + | </math> | ||
| + | |||
| + | |||
| + | <math> | ||
| + | f+e^{r\cdot t + C}\cdot f = e^{r\cdot t + C} | ||
| + | </math> | ||
| + | |||
| + | <math> | ||
| + | f\cdot(e^{r\cdot t + C}+1) = e^{r\cdot t + C} | ||
| + | </math> | ||
| + | |||
| + | <math> | ||
| + | f = \frac{e^{r\cdot t + C}}{(e^{r\cdot t + C}+1)}=\frac{1}{1+\frac{1}{e^{r\cdot t + C}}} | ||
| + | </math> | ||
| + | |||
| + | Die Lösungsfunktion der logistischen DGLG lautet nun: | ||
| + | |||
| + | <math> | ||
| + | f(t)=\frac{1}{1+e^{-r\cdot t +C}} | ||
| + | </math> | ||
| + | <br><br> | ||
| + | [[:Bild:Logistisches_wachstum.png|<b>Graph der Funktion in besserer Auflösung!</b>]]<br> | ||
| + | [[Datei:Logistisches wachstum.png|miniatur|x150px|Graph der logistischen Funktion]] | ||
| + | <br> | ||
| + | Die Integrationskonstante <math>\,C </math> kann mittels der Anfangsbedingung <math>\,A(0/f_{0})</math> ermittelt werden. | ||
| + | |||
| + | <math> | ||
| + | f_{0}=\frac{1}{1+e^{-r\cdot 0 +C}}=\frac{1}{1+e^{C}} | ||
| + | </math> | ||
| + | |||
| + | <math> | ||
| + | f_{0}\cdot(1+e^{C})=1 | ||
| + | </math> | ||
| + | |||
| + | <math> | ||
| + | 1+e^{C}=\frac{1}{f_{0}} | ||
| + | </math> | ||
| + | |||
| + | <math> | ||
| + | e^{C}=\frac{1}{f_{0}}-1 | ||
| + | </math> | ||
| + | |||
| + | <math> | ||
| + | C=ln(\frac{1}{f_{0}}-1) | ||
| + | </math> | ||
| + | |||
| + | mit <math>\,f_{0}<0</math>, da die maximale Population auf <math>\,1 </math> normiert ist. <br><br> | ||
| + | |||
| + | Die komplette Lösung lautet nun: | ||
| + | |||
| + | <math> | ||
| + | f(t)=\frac{1}{1+e^{-r\cdot t +ln(\frac{1}{f_{0}}-1)}} | ||
| + | </math> | ||
| + | |||
| + | Link: | ||
| + | * [http://www.emath.de/Referate/Logistisches-Wachstum.pdf Chr. Bruns, Logistisches Wachstum, Facharbeit] | ||
[[Diskret - kontinuierlich|Zurück zum Lernpfad]] | [[Diskret - kontinuierlich|Zurück zum Lernpfad]] | ||
Version vom 22. August 2011, 12:43 Uhr
Als logistische Gleichung wird eine Differenzengleichung der Form 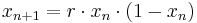 bezeichnet. Sie wird verwendet, um ein Wachstum mit Schranke zu modellieren. Die
bezeichnet. Sie wird verwendet, um ein Wachstum mit Schranke zu modellieren. Die 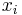 liegen im Intervall zwischen
liegen im Intervall zwischen 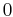 und
und 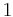 und beschreiben die Größe einer Population prozentuell. In der Konstanten
und beschreiben die Größe einer Population prozentuell. In der Konstanten  sind Parameter wie Wachstumsrate, Sterblichkeit und Ähnliches zusammengefasst. Die zugehörige Differentialgleichung (DGLG) sieht folgendermaßen aus:
sind Parameter wie Wachstumsrate, Sterblichkeit und Ähnliches zusammengefasst. Die zugehörige Differentialgleichung (DGLG) sieht folgendermaßen aus:
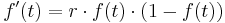 Diese DGLG kann analytisch gelöst werden, zur Vereinfachung wird statt
Diese DGLG kann analytisch gelöst werden, zur Vereinfachung wird statt 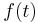
 geschrieben:
geschrieben:
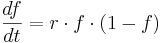
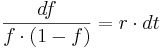
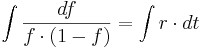

Mittels Partialbruchzerlegung ermittelt man für  und
und 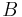 jeweils den Wert
jeweils den Wert 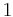 .
.
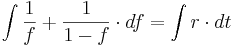
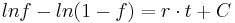
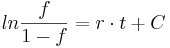
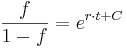
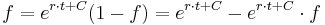
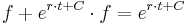
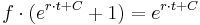

Die Lösungsfunktion der logistischen DGLG lautet nun:

Graph der Funktion in besserer Auflösung!
Die Integrationskonstante  kann mittels der Anfangsbedingung
kann mittels der Anfangsbedingung 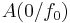 ermittelt werden.
ermittelt werden.
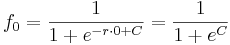
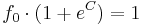
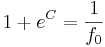
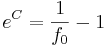
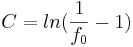
mit  , da die maximale Population auf
, da die maximale Population auf 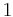 normiert ist.
normiert ist.
Die komplette Lösung lautet nun:
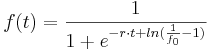
Link:


