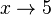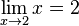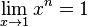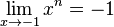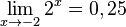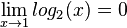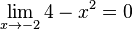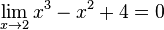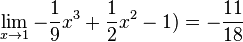Grenzwert: Unterschied zwischen den Versionen
| Zeile 54: | Zeile 54: | ||
# <math> f: x \rightarrow 2^x</math> für <math> x \rightarrow \infty</math> | # <math> f: x \rightarrow 2^x</math> für <math> x \rightarrow \infty</math> | ||
# <math> f: x \rightarrow log_2 (x)</math> für <math> x \rightarrow \infty</math> | # <math> f: x \rightarrow log_2 (x)</math> für <math> x \rightarrow \infty</math> | ||
| − | # <math> f: x \rightarrow log_2 (x)</math> für <math> x \rightarrow | + | # <math> f: x \rightarrow log_2 (x)</math> für <math> x \rightarrow 0</math> |
| − | + | ||
# <math> f: x \rightarrow 4 - x^2</math> für <math> x \rightarrow \infty</math> | # <math> f: x \rightarrow 4 - x^2</math> für <math> x \rightarrow \infty</math> | ||
# <math> f: x \rightarrow x^3 - x^2 + 4</math> für <math> x \rightarrow - \infty</math> | # <math> f: x \rightarrow x^3 - x^2 + 4</math> für <math> x \rightarrow - \infty</math> | ||
| Zeile 73: | Zeile 72: | ||
# <math>\lim_{x \to -\infty}2^x = 0</math> | # <math>\lim_{x \to -\infty}2^x = 0</math> | ||
# <math>\lim_{x \to \infty}log_2 (x) = \infty</math> | # <math>\lim_{x \to \infty}log_2 (x) = \infty</math> | ||
| − | # <math>\lim_{x \to | + | # <math>\lim_{x \to 0}log_2 (x) = -\infty</math> |
# <math>\lim_{x \to -\infty}4 - x^2 = -\infty</math> | # <math>\lim_{x \to -\infty}4 - x^2 = -\infty</math> | ||
# <math>\lim_{x \to \infty}x^3 - x^2 + 4 = \infty</math> | # <math>\lim_{x \to \infty}x^3 - x^2 + 4 = \infty</math> | ||
| Zeile 79: | Zeile 78: | ||
# <math>\lim_{x \to -\infty}-\frac{1}{9}x^3 + \frac{1}{2}x^2-1) = \infty</math> | # <math>\lim_{x \to -\infty}-\frac{1}{9}x^3 + \frac{1}{2}x^2-1) = \infty</math> | ||
}} | }} | ||
| + | |||
| + | {{Arbeiten| | ||
| + | NUMMER=2| | ||
| + | ARBEIT= | ||
| + | Bestimme die Grenzwerte gegen <math> -\infty</math> oder <math> \infty</math> folgender Funktionen | ||
| + | |||
| + | # <math> f: x \rightarrow x</math> für <math> x \rightarrow 2</math> | ||
| + | # <math> f: x \rightarrow x^2</math> für <math> x \rightarrow -3</math> | ||
| + | # <math> f: x \rightarrow x^n</math> für <math> x \rightarrow 1</math> | ||
| + | # <math> f: x \rightarrow x^n</math> für <math> x \rightarrow -1</math> wenn n ungerade | ||
| + | # <math> f: x \rightarrow x^n</math> für <math> x \rightarrow -1</math> wenn n gerade | ||
| + | # <math> f: x \rightarrow 2^x</math> für <math> x \rightarrow 5</math> | ||
| + | # <math> f: x \rightarrow 2^x</math> für <math> x \rightarrow -2</math> | ||
| + | # <math> f: x \rightarrow log_2 (x)</math> für <math> x \rightarrow 1</math> | ||
| + | # <math> f: x \rightarrow log_2 (x)</math> für <math> x \rightarrow 1024</math> | ||
| + | # <math> f: x \rightarrow 4 - x^2</math> für <math> x \rightarrow -2</math> | ||
| + | # <math> f: x \rightarrow x^3 - x^2 + 4</math> für <math> x \rightarrow 2</math> | ||
| + | # <math>f:x \rightarrow -\frac{1}{9}x^3 + \frac{1}{2}x^2-1</math> für <math> x \rightarrow 0</math> | ||
| + | # <math>f:x \rightarrow -\frac{1}{9}x^3 + \frac{1}{2}x^2-1</math> für <math> x \rightarrow 1</math> | ||
| + | }} | ||
| + | |||
| + | Falls du Hilfe brauchst, öffne GeoGebra,gib die Funktionsterme ein und betrachte den Graphen. | ||
| + | |||
| + | {{Lösung versteckt| | ||
| + | # <math>\lim_{x \to 2}x = 2</math> | ||
| + | # <math>\lim_{x \to -3}x^2 = 9</math> | ||
| + | # <math>\lim_{x \to 1}x^n = 1</math> | ||
| + | # <math>\lim_{x \to -1}x^n = -1</math> | ||
| + | # <math>\lim_{x \to -1}x^n = 1</math> | ||
| + | # <math>\lim_{x \to 5}2^x = \infty =32</math> | ||
| + | # <math>\lim_{x \to -2}2^x = 0,25</math> | ||
| + | # <math>\lim_{x \to 1}log_2 (x) = 0</math> | ||
| + | # <math>\lim_{x \to 1024)log_2 (x) = 10</math> | ||
| + | # <math>\lim_{x \to -2}4 - x^2 = 0</math> | ||
| + | # <math>\lim_{x \to 2}x^3 - x^2 + 4 = 0</math> | ||
| + | # <math>\lim_{x \to 0}-\frac{1}{9}x^3 + \frac{1}{2}x^2-1) = -1</math> | ||
| + | # <math>\lim_{x \to 1}-\frac{1}{9}x^3 + \frac{1}{2}x^2-1) = -\frac{11}{18}</math> | ||
| + | }} | ||
| + | |||
| + | |||
| + | ---- | ||
| + | |||
| + | zurück zu [[Funktionen_Einstieg/Eigenschaften von Funktionen|Eigenschaften von Funktionen]] | ||
Version vom 2. Januar 2012, 17:54 Uhr
|
Zuerst vereinbaren wir: sehr große positve Zahlen sind solche positive Zahlen mit großem Betrag (z.B. 1000000) sehr kleine positive Zahlen sind solche positive Zahlen mit kleinem Betrag (z.B. 0,0000001) Grenzwerte von Funktionen spiegeln das Verhalten im Unendlichen wieder oder, falls wir x gegen einen anderen Wert als unendlich laufen lassen, das entsprechende Verhalten. Beispiel: Wir betrachten die Funktion Wir wollen x gegen unendlich und gegen minus unendlich laufen lassen. Wir schreiben für x gegen unendlich: und für x gegen minus unendlich: Da in beiden Fällen die Funktionswerte immer größer werden und über alle Grenzen wachsen, ist dann Ein Plus-oder Minuszeichen rechts hochgestellt an einer Zahl bei Grenzwerten bedeutet Annäherung von rechts – bzw. von links her an die Zahl
Es ist dann
|
Auf dieser Seite sind zwei Beispiele ausführlich erklärt.
Auf den folgenden Seiten sind zum Thema Grenzwert weitere Erklärungen und Beispiele zu finden:
- Grenzwert an einer Stelle
- Beispiel mit Grenzwert und ohne Grenzwert
- Beispiele: Identische Funktion, konstante Funktion, Betragsfunktion, trigonometrische Funktionen, Potenz-, Exponential- und Logarithmusfunktionen
|
Bestimme die Grenzwerte gegen
|
Falls du Hilfe brauchst, öffne GeoGebra,gib die Funktionsterme ein und betrachte den Graphen.
|
Bestimme die Grenzwerte gegen
|
Falls du Hilfe brauchst, öffne GeoGebra,gib die Funktionsterme ein und betrachte den Graphen.
zurück zu Eigenschaften von Funktionen
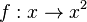
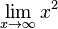
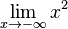
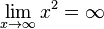 und
und 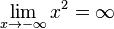
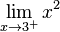 heißt: x ist bei der Annäherung größer als 3, also z.B. 3,1 ; 3,01 ; 3,001 usw.
heißt: x ist bei der Annäherung größer als 3, also z.B. 3,1 ; 3,01 ; 3,001 usw.
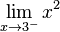 heißt: x ist bei der Annäherung kleiner als 3, also z.B. 2,9 ; 2,99 ; 2,999 usw.
heißt: x ist bei der Annäherung kleiner als 3, also z.B. 2,9 ; 2,99 ; 2,999 usw.
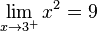 bzw.
bzw. 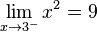 .
.
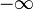 oder
oder  folgender Funktionen
folgender Funktionen
 für
für 
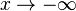
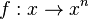 für
für 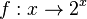 für
für  für
für 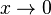
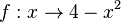 für
für 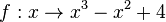 für
für 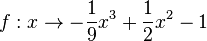 für
für 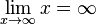
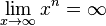
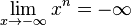
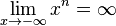
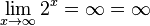
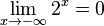
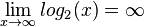
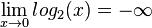

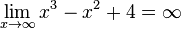
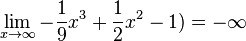
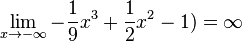
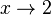


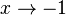 wenn n ungerade
wenn n ungerade