Rationale Funktionen senkrechte Asymptoten: Unterschied zwischen den Versionen
Aus Medienvielfalt-Wiki
(Die Seite wurde neu angelegt: „{{Merke|Ist an einer Definitionslücke <math>x_0</math> einer gebrochen-rationalen Funktion <math>f</math> <math>\lim_{x \to x_0}\left| f(x) \right|=\infty</math…“) |
|||
| Zeile 1: | Zeile 1: | ||
| + | Die Funktion <math>f:x\rightarrow \frac{x-2}{x^2-1}</math> hat die Definitionslücken <math> x = -1</math> und <math> x = 1</math>. | ||
| + | |||
| + | Es ist <math>\lim_{x \to -1}\left| f(x) \right|=\infty</math>, da z(-1) = 1 ist. <math> x = -1 </math> ist Polstelle und die Gerade <math> x = -1 </math> ist senkrechte Asymptote für den Graphen von f. | ||
| + | |||
| + | Ebenso ist <math>\lim_{x \to 1}\left| f(x) \right|=\infty</math>, da z(1) = 1 ist. <math> x = 1 </math> ist Polstelle und die Gerade <math> x = 1 </math> ist senkrechte Asymptote für den Graphen von f. | ||
| + | |||
{{Merke|Ist an einer Definitionslücke <math>x_0</math> einer gebrochen-rationalen Funktion <math>f</math> | {{Merke|Ist an einer Definitionslücke <math>x_0</math> einer gebrochen-rationalen Funktion <math>f</math> | ||
| Zeile 6: | Zeile 12: | ||
Die Gerade mit der Gleichung <math>x = x_0</math> ist '''senkrechte Asymptote''' des Graphen von f. }} | Die Gerade mit der Gleichung <math>x = x_0</math> ist '''senkrechte Asymptote''' des Graphen von f. }} | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
Aktuelle Version vom 5. März 2013, 20:55 Uhr
Die Funktion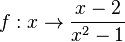 
hat die Definitionslücken 
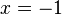und 
.
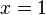Es ist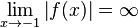 
, da z(-1) = 1 ist. 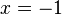ist Polstelle und die Gerade 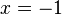ist senkrechte Asymptote für den Graphen von f.
Ebenso ist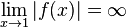 
, da z(1) = 1 ist. 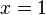ist Polstelle und die Gerade 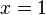ist senkrechte Asymptote für den Graphen von f.
 Merke
Ist an einer Definitionslücke
dann ist die Definitionslücke Die Gerade mit der Gleichung |
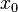einer gebrochen-rationalen Funktion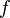 
,
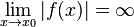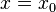ist senkrechte Asymptote des Graphen von f.
