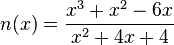Rationale Funktionen Nullstellen: Unterschied zwischen den Versionen
Aus Medienvielfalt-Wiki
| (3 dazwischenliegende Versionen von einem Benutzer werden nicht angezeigt) | |||
| Zeile 1: | Zeile 1: | ||
| − | Die Nullstellen einer gebrochen-rationalen Funktion findet man, indem man den Zähler der Funktion betrachtet. | + | Die Nullstellen einer gebrochen-rationalen Funktion findet man, indem man den Zähler der Funktion betrachtet, denn ein Bruch hat den Wert <math>0</math>, wenn der Zähler den Wert <math>0</math> hat. |
<math>f:x \rightarrow \frac{2-x}{x^2}</math> hat den Funktionswert <math>0</math>, wenn der Zähler <math> 2-x = 0</math> ist. | <math>f:x \rightarrow \frac{2-x}{x^2}</math> hat den Funktionswert <math>0</math>, wenn der Zähler <math> 2-x = 0</math> ist. | ||
| Zeile 8: | Zeile 8: | ||
}} | }} | ||
| − | {{ | + | {{Arbeiten|NUMMER=1| |
| + | ARBEIT= | ||
Ordne die Nullstellen und die angegebenen Funktionen <math> f: x \rightarrow f(x)</math> richtig zu! | Ordne die Nullstellen und die angegebenen Funktionen <math> f: x \rightarrow f(x)</math> richtig zu! | ||
}} | }} | ||
| Zeile 27: | Zeile 28: | ||
| <math>f(x) = \frac{x^2-64x}{x^2+64}</math> || <math>x_1 = -8; x_2 = 8</math> | | <math>f(x) = \frac{x^2-64x}{x^2+64}</math> || <math>x_1 = -8; x_2 = 8</math> | ||
|} | |} | ||
| + | </div> | ||
| + | |||
| + | {{Arbeiten|NUMMER=2| | ||
| + | ARBEIT= | ||
| + | Ermittle jeweils die Nullstellen der Funktion: | ||
| + | |||
| + | a) <math>f</math> mit <math>f(x) = \frac{13-x}{(x-1)^2}</math> | ||
| + | |||
| + | b) <math>g</math> mit <math>g(x) = \frac{16-x^2}{x^2+1}</math> | ||
| + | |||
| + | c) <math>h</math> mit <math>h(x) = \frac{16}{x^2-1}</math> | ||
| + | |||
| + | d) <math>k</math> mit <math>k(x) = \frac{x^2+3x+2}{(x-3)(x-2)}</math> | ||
| + | |||
| + | e) <math>l</math> mit <math>l(x) = \frac{x^2-5x+6}{(x^2+7)}</math> | ||
| + | |||
| + | f) <math>m</math> mit <math>m(x) = \frac{x^3+x^2-6x}{x^4+1}</math> | ||
| + | |||
| + | g) <math>n</math> mit <math>n(x) = \frac{x^3+x^2-6x}{x^2+4x+4}</math> | ||
| + | }} | ||
| + | |||
| + | {{Lösung versteckt|1= | ||
| + | |||
| + | a) x = 13 | ||
| + | |||
| + | b) x = -4 ; x = 4 | ||
| + | |||
| + | c) keine | ||
| + | |||
| + | d) x = -2; x= -1 | ||
| + | |||
| + | e) x= 2; x = 3 | ||
| + | |||
| + | f) x = -3; x = 0; x = 2 | ||
| + | |||
| + | g) x = -3; x = 0; (x = 2 muss näher untersucht werden, da 2 auch Nullstelle des Nenners ist!) | ||
| + | }} | ||
Aktuelle Version vom 4. April 2013, 10:06 Uhr
Die Nullstellen einer gebrochen-rationalen Funktion findet man, indem man den Zähler der Funktion betrachtet, denn ein Bruch hat den Wert 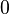 , wenn der Zähler den Wert
, wenn der Zähler den Wert 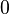 hat.
hat.
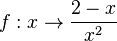 hat den Funktionswert
hat den Funktionswert 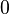 , wenn der Zähler
, wenn der Zähler 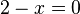 ist.
ist.
|
|
|
Ordne die Nullstellen und die angegebenen Funktionen |
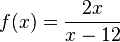 |
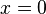
|
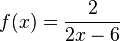 |
keine Nullstelle |
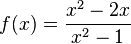 |
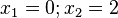
|
 |
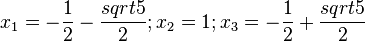
|
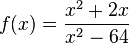 |
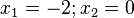
|
 |
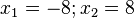
|
|
Ermittle jeweils die Nullstellen der Funktion: a) b) c) d) e) f) g) |
a) x = 13
b) x = -4 ; x = 4
c) keine
d) x = -2; x= -1
e) x= 2; x = 3
f) x = -3; x = 0; x = 2
g) x = -3; x = 0; (x = 2 muss näher untersucht werden, da 2 auch Nullstelle des Nenners ist!)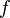 mit
mit 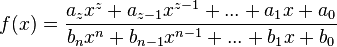 hat für
hat für 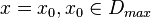 den Funktionswert Null, wenn das Zählerpolynom
den Funktionswert Null, wenn das Zählerpolynom 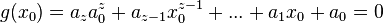 ist.
ist.
 richtig zu!
richtig zu!
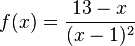
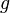 mit
mit 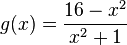
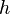 mit
mit 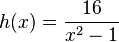
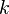 mit
mit 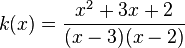
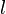 mit
mit 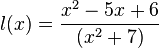
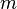 mit
mit 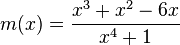
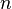 mit
mit