Rationale Funktionen hebbare Definitionslücken: Unterschied zwischen den Versionen
| (7 dazwischenliegende Versionen von einem Benutzer werden nicht angezeigt) | |||
| Zeile 5: | Zeile 5: | ||
}} | }} | ||
| − | Die neue Funktion <math>\tilde f:x\rightarrow \frac{1}{x+2}</math> ist für <math> x = 1 </math> mit dem Funktiionswert <math>\tilde f(1) = \frac{1}{3}</math> definiert. Man kann also die Funktion <math> f</math> in die hebbare Definitionslücke fortsetzen. Nimmt man den Funktionswert von <math>\tilde f(1)=\frac{1}{3}</math>, dann hat man die Funktion sogar stetig fortgesetzt. | + | Die neue Funktion <math>\tilde f:x\rightarrow \frac{1}{x+2}</math> ist für <math> x = 1 </math> mit dem Funktiionswert <math>\tilde f(1) = \frac{1}{3}</math> definiert. Man kann also die Funktion <math> f</math> in die hebbare Definitionslücke fortsetzen. Nimmt man den Funktionswert von <math>\tilde f(1)=\frac{1}{3}</math>, dann hat man die Funktion sogar stetig fortgesetzt. Die Funktion <math>\tilde f</math> ist identisch mit der Funktion <math>f</math>, nur dass sie auch noch für <math>x=1</math> definiert ist. |
| + | |||
| + | {{Aufgabe|1= | ||
| + | Im folgenden Applet wird die Funktion <math>f:x \rightarrow f(x) = \frac{(x+3)(x-2)^2}{(x-2)^n} </math> dargestellt . <br> | ||
| + | Variiere mit dem Schieberegler den Wert des Exponenten der Nennerpotenz. <br> | ||
| + | |||
| + | Beobachte die Veränderungen für <math>x=2</math> beim Variieren von n. Formuliere deine Beobachtung. | ||
| + | <center> | ||
| + | <ggb_applet width="532" height="492" version="4.2" ggbBase64="UEsDBBQACAAIAD15hkIAAAAAAAAAAAAAAAAWAAAAZ2VvZ2VicmFfamF2YXNjcmlwdC5qc0srzUsuyczPU0hPT/LP88zLLNHQVKiuBQBQSwcI1je9uRkAAAAXAAAAUEsDBBQACAAIAD15hkIAAAAAAAAAAAAAAAAMAAAAZ2VvZ2VicmEueG1svVdtb9s2EP6c/oqDPiVbbIsi9eLCbtFuKFYg7YqlG4Z9GEBLtM1GlgSRsuWiP35HUpKVNAm6tqhhm6R4vIf33AupxfN2l8Ne1EqWxdIjU98DUaRlJovN0mv0epJ4z589WWxEuRGrmsO6rHdcLz02DbzTOhxNSWgWywy1JKlPw0hMwnngT1gQigkXiT/ha5/TMGCCJdwDaJV8WpRv+U6oiqfiOt2KHb8qU66tzq3W1dPZ7HA4THv0aVlvZpvNatqqzAPceaGWXtd5iupuLTpQKx74Ppn9/ebKqZ/IQmlepMIDY1Ujnz05WxxkkZUHOMhMb5deyNCMrZCbLZoZBTiYGaEKba1EquVeKFw6Glqb9a7yrBgvzPyZ60E+mONBJvcyE7Vhec4SMvoilWUtRaE7WdJhznpti70UB6fW9Cxi4oEuy3zFjUY0Ej4BNoFrKMAn2wndmHXDyA1j2xC/e5qYv7kZROhUqeQqF0tvzXOFPMliXaOPhrHSx1xY1O7ByUZySXFefkRh6iNxjli7vUvzi/DH/I7RkSlkhKrr5lFQN3/CHBBDGnw5IhuRZ1kI/MCHS9MQ1wTYRJGb8t0zZNU2gWuYa0Inw9xy5kSZk2FOhtFvo7U3Mfg/pAbfhHlyZHIPZhA+4MjH4sd57kvsJOEIE6Hs1/4+Q6SPmXkX8W7sfA1gxH60icyfx9/dSMzPS+qzy4jdDxr792aIa0nX/hDuF7O++C26DYHaGtmuUmixU2aLdA6hrWEEQkzYKMbUC4HMsYlNVQyAhMBCHJIEItPGQE0hZEAhASNHKNiMDRP8Y7FVFkGIyszT2LdVEyiDkAKxic4AeQBbLJCVgKJEGEKIiww8CYwKGgGLcEQTYLhHUydiU60pLsQxwgdACVCzmMQQRBAFEJtaQ5gpQVFido9aA4h8iCz5WG2w0rgqg0sSoMYgzL6qVHIgeCvyqufJUimLqtG36Et3Wd/VZTW40UpnZXrzcqC7mxFc6bEYHlKno9AdWrdOyrNFzlcix/vEtYkFgD3PTXmxCOuy0NDHQeCebWpebWWqroXWuErBB77nV1yL9hVKqx7bQtsDfCGaNJeZ5MVfGChGhVEIw3luSkR/njNMX4uSlmWdXR8VRg+0/4i6xIJJkmnsUxKwuftgph+7qZhMWTwnUfcx1THlJuwpndLRJ0jQB8eH5yy22A+28Vaonv9NLbNx/7V6WebZwHVVykL/wivd1PZyhnuojVEvik0uLLe25uM1J71Zle21I5U6Xe+PFY58h7/a/FLmZQ2YlUEYokDXrlxrZczGBinfyvhWwu+9JLNhnswDK2HblWutFLrdba0zlPRWEr+HkcrWG1Q+DksbM0uv9aAppL5yIwxRmd50phK34G2zW2G8DTGMAr9Kd8Vz19nbMORemON3gVnM7gTi4kbUhchduBXo8KZslIv/IYbPFo0S77jeviiyP8QGM/cdNwVUI5oTtYguH0Uqd7jQPe8o5sb9f+Lu3dNMbGrRyfPc3pqdA+ysPw7+zx5bVa/qcve62L/H2Lqz1cWst2eh0lpWJoJhhRX9RpyiNJOK43mQjdeh8QqtSE1dQuq04dUD3uhtWduLMSY3HqzwoqpljvUUg9ZkdS52eCUGbUO3aHailungssLetnGDTWcD631t3AXl6gNWoDtuPjGJ0w8EN/C82nLsTUkXwvwo6ls0WW1vyqwD7uRUbi73sJOu9O54a2+ZfKXKvNH4doNuKU5vN25jXYnCK5V5d2ptycDOER/Fc9Nby3ZELvIlP2Ik3Q6LU5JprJs3+MagbCXQXc7bzm8yy0Qx7JYXGEnWH1gBKxfmlRAuQ4aFFRpva80oBjq3GAe1VY1YRklH8BqPh9YcIuftBSzh/LyFn4FewE+AvQkEF/+eBxcXMDsNiwt3iNx29ropbLB4J8Vf41m8eD/gW/9R3/6+XiuhjTsmofNGFDzm+nsdQB9zwJjG2Tg97KHWve0++w9QSwcITiMD1swFAACdDwAAUEsBAhQAFAAIAAgAPXmGQtY3vbkZAAAAFwAAABYAAAAAAAAAAAAAAAAAAAAAAGdlb2dlYnJhX2phdmFzY3JpcHQuanNQSwECFAAUAAgACAA9eYZCTiMD1swFAACdDwAADAAAAAAAAAAAAAAAAABdAAAAZ2VvZ2VicmEueG1sUEsFBgAAAAACAAIAfgAAAGMGAAAAAA==" showResetIcon = "true" enableRightClick = "false" errorDialogsActive = "true" enableLabelDrags = "false" showMenuBar = "false" showToolBar = "false" showToolBarHelp = "false" showAlgebraInput = "false" useBrowserForJS = "true" allowRescaling = "true" /> | ||
| + | </center> | ||
| + | }} | ||
| + | |||
| + | {{Lösung versteckt|1= | ||
| + | Für n = 4 ist <math>x=2</math> nicht hebbare Definitionslücke. Der Graph hat an der Stelle <math>x=2</math> eine Polstelle ohne Vorzeichenwechsel. | ||
| + | |||
| + | Für n = 3 ist <math>x=2</math> nicht hebbare Definitionslücke. Der Graph hat an der Stelle <math>x=2</math> eine Polstelle mit Vorzeichenwechsel. | ||
| + | |||
| + | Für n = 2 ist die Definitionslücke <math>x=2</math> hebbar. | ||
| + | |||
| + | Ebenso sieht der Graph für n = 1 "durchgezeichnet" aus, <math>x=2</math> ist eine hebbare Definitionslücke. | ||
| + | |||
| + | Tatsächlich ist aber für n = 2 oder n = 1 an der Stelle <math>x = 2</math> ein Loch. Die Funktion <math>f</math> ist dort nicht definiert! Man kann <math>f</math> aber in <math>x = 2</math> fortsetzen, dies macht die Funktion <math>\tilde f</math>.<br> | ||
| + | Für n = 2 würde man am Graph den Wert <math>\tilde f(2)=5</math> ablesen.<br> | ||
| + | Für n = 1 würde man am Graph den Wert <math>\tilde f(2)=0</math> ablesen. | ||
| + | }} | ||
{{Arbeiten|NUMMER=1| | {{Arbeiten|NUMMER=1| | ||
| Zeile 23: | Zeile 47: | ||
f) <math>f</math> mit <math>f(x) = \frac{x^3+x^2-6x}{x-3}</math> | f) <math>f</math> mit <math>f(x) = \frac{x^3+x^2-6x}{x-3}</math> | ||
| + | g) <math>f</math> mit <math>f(x) = \frac{x^3+x^2-6x}{x^2-5x+6}</math> | ||
| − | |||
}} | }} | ||
{{Lösung versteckt|1= | {{Lösung versteckt|1= | ||
| − | a) | + | a) <math> x = 2</math> ist eine Definitionslücke; wegen <math>\frac{x^2-4}{x-2}=\frac{(x-2)(x+2)}{x-2}=x+2</math> ist <math>x=2</math> hebbare Definitionslücke mit <math>\tilde f(2)=4</math>. |
| − | b) | + | b) <math> x = 0; x = 2</math> sind Definitionslücken; wegen <math>\frac{4x}{x^2-2x}=\frac{4x}{x(x-2)}=\frac{4}{x-2}</math> ist <math>x=0</math> hebbare Definitionslücke mit <math>\tilde f(0)=-2</math>. |
| − | c) | + | c) <math> x = -7</math> ist Definitionslücke; da man den Bruchterm nicht kürzen kann, ist <math> x = -7</math> keine hebbare Definitionslücke. |
| − | d) | + | d) <math> x = 3</math> ist Definitionslücke; da man den Bruchterm nicht kürzen kann, ist <math> x = 3</math> keine hebbare Definitionslücke. |
| − | e) | + | e) <math> x = -2</math> ist Definitinoslücke; wegen <math>f(x) = \frac{x^3+x^2-6x}{x^2+4x+4}=\frac{x(x+2)(x-3)}{(x+2)^2}=\frac{x(x-3)}{x+2}</math> ist <math>x=-2</math> weiterhin Definitionslücke und nicht hebbar. |
| − | f) | + | f) <math> x = 3</math> ist Definitionslücke; wegen <math>f(x) = \frac{x^3+x^2-6x}{x-3}=\frac{x(x+2)(x-3)}{x-3}=x(x+2)</math> ist <math>x=3</math> eine hebbare Definitionslücke mit <math>\tilde f(3)=15</math>. |
| + | |||
| + | g) <math> x = 2; x = 3</math> sind Definitionslücken; wegen <math>\frac{x^3+x^2-6x}{x^2-5x+6}=\frac{x(x+2)(x-3)}{(x-2)(x-3)}=\frac{x(x+2)}{x-2}</math> eine hebbare Definitionslücke mit <math>\tilde f(3)=15</math>. | ||
}} | }} | ||
| + | |||
| + | {{Arbeiten|NUMMER=2| | ||
| + | ARBEIT= | ||
| + | Ordne die hebbare bzw. nicht hebbare Definitionslücke und die angegebene Funktion <math>f:x \rightarrow f(x)</math> richtig zu! | ||
| + | }} | ||
| + | |||
| + | <div class="zuordnungs-quiz"> | ||
| + | |||
| + | {| | ||
| + | | <math>f(x) = \frac{2x}{x-12}</math> || x=12 ist nicht hebbare Definitionslücke. | ||
| + | |- | ||
| + | | <math>f(x) = \frac{x-3}{2x^2-6x}</math> || x=3 ist hebbare Definitionslücke. | ||
| + | |- | ||
| + | | <math>f(x) = \frac{x^2-x}{x^2-1}</math> || x=1 ist hebbare Definitionslücke. | ||
| + | |- | ||
| + | | <math>f(x) = \frac{x^2-2x+1}{x^2-3x+2}</math> || x=2 ist nicht hebbare Definitionslücke. | ||
| + | |- | ||
| + | | <math>f(x) = \frac{x^2-8x}{x^2-64}</math> || x=8 ist hebbare Definitionslücke. | ||
| + | |- | ||
| + | | <math>f(x) = \frac{x^2-5x+4}{x^2-7x+12}</math> || x=3 ist nicht hebbare Definitionslücke. | ||
| + | |} | ||
| + | </div> | ||
Aktuelle Version vom 6. April 2013, 15:27 Uhr
Die Funktion Fehler beim Parsen(Unbekannte Funktion „\fra“): f:x\rightarrow \fra{x-1}{x^2+x-2}
ist an den Nullstellen des Nenners, also für
nicht erklärt. Vereinfacht man den Funktionsterm
so ist der gekürzte Term
für
erklärt mit dem Wert
. Man sagt, dass
eine hebbare Definitionslücke ist.
|
Ist |
Die neue Funktion 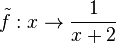 ist für
ist für 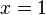 mit dem Funktiionswert
mit dem Funktiionswert  definiert. Man kann also die Funktion
definiert. Man kann also die Funktion 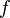 in die hebbare Definitionslücke fortsetzen. Nimmt man den Funktionswert von
in die hebbare Definitionslücke fortsetzen. Nimmt man den Funktionswert von  , dann hat man die Funktion sogar stetig fortgesetzt. Die Funktion
, dann hat man die Funktion sogar stetig fortgesetzt. Die Funktion 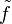 ist identisch mit der Funktion
ist identisch mit der Funktion 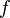 , nur dass sie auch noch für
, nur dass sie auch noch für 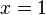 definiert ist.
definiert ist.
|
Im folgenden Applet wird die Funktion Beobachte die Veränderungen für
|
|
Gib jeweils für die Funktion a) b) c) d) e) f) g) |
|
Ordne die hebbare bzw. nicht hebbare Definitionslücke und die angegebene Funktion |
x=3 ist hebbare Definitionslücke.
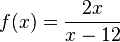
x=8 ist hebbare Definitionslücke.
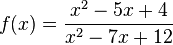
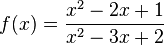
x=1 ist hebbare Definitionslücke.
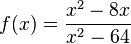
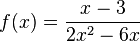
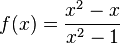 x=3 ist nicht hebbare Definitionslücke.x=12 ist nicht hebbare Definitionslücke.x=2 ist nicht hebbare Definitionslücke.
x=3 ist nicht hebbare Definitionslücke.x=12 ist nicht hebbare Definitionslücke.x=2 ist nicht hebbare Definitionslücke.
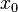 eine Nullstelle des Zählers und des Nenners der gebrochen-rationalen Funktion
eine Nullstelle des Zählers und des Nenners der gebrochen-rationalen Funktion 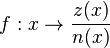 und existiert der Grenzwert
und existiert der Grenzwert  , so nennt man
, so nennt man 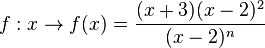 dargestellt .
dargestellt . 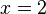 beim Variieren von n. Formuliere deine Beobachtung.
beim Variieren von n. Formuliere deine Beobachtung.
 ablesen.
ablesen.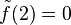 ablesen.
ablesen.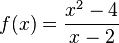
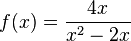
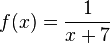
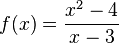
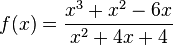
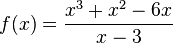

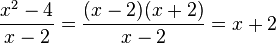 ist
ist  .
.
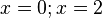 sind Definitionslücken; wegen
sind Definitionslücken; wegen 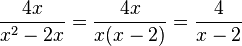 ist
ist 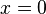 hebbare Definitionslücke mit
hebbare Definitionslücke mit 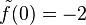 .
.
 ist Definitionslücke; da man den Bruchterm nicht kürzen kann, ist
ist Definitionslücke; da man den Bruchterm nicht kürzen kann, ist  ist Definitionslücke; da man den Bruchterm nicht kürzen kann, ist
ist Definitionslücke; da man den Bruchterm nicht kürzen kann, ist 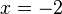 ist Definitinoslücke; wegen
ist Definitinoslücke; wegen 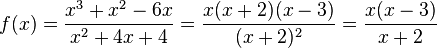 ist
ist  ist
ist  .
.
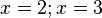 sind Definitionslücken; wegen
sind Definitionslücken; wegen 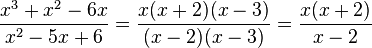 eine hebbare Definitionslücke mit
eine hebbare Definitionslücke mit  richtig zu!
richtig zu!

