Eigenschaften von Funktionen: Unterschied zwischen den Versionen
| Zeile 63: | Zeile 63: | ||
d.h. für alle <math> x_1,x_2 \in [a;b]</math> gilt: <math>x_1 < x_2 \Rightarrow f(x_1) > f(x_2)</math> | d.h. für alle <math> x_1,x_2 \in [a;b]</math> gilt: <math>x_1 < x_2 \Rightarrow f(x_1) > f(x_2)</math> | ||
}} | }} | ||
| + | |||
| + | {{Arbeiten|NUMMER=3|ARBEIT= | ||
| + | |||
| + | Teste dich! Klicke im folgenden Quiz auf die richtigen Zuordnungen! | ||
| + | }} | ||
| + | |||
| + | <div class="multiplechoice-quiz"> | ||
| + | |||
| + | [[Bild:Monotonie_f1.jpg|200px]] | ||
| + | (streng monoton steigend) (!streng monoton fallend) (!weder noch) | ||
| + | |||
| + | [[Bild:Monotonie_f2.jpg|200px]] | ||
| + | (!streng monoton steigend) (streng monoton fallend) (!weder noch) | ||
| + | |||
| + | [[Bild:Monotonie_f3.jpg|200px]] | ||
| + | (!streng monoton steigend) (streng monoton fallend) (!weder noch) | ||
| + | |||
| + | [[Bild:Monotonie_f6.jpg|200px]] | ||
| + | (streng monoton steigend) (!streng monoton fallend) (!weder noch) | ||
| + | |||
| + | [[Bild:Monotonie_f4.jpg|200px]] | ||
| + | (!streng monoton steigend) (!streng monoton fallend) (weder noch) | ||
| + | |||
| + | [[Bild:Monotonie_f5.jpg|200px]] | ||
| + | (!streng monoton steigend) (!streng monoton fallend) (weder noch) | ||
| + | |||
| + | [[Bild:Monotonie_f7.jpg|200px]] | ||
| + | (streng monoton steigend) (!streng monoton fallend) (!weder noch) | ||
| + | |||
| + | </div> | ||
=Grenzwert= | =Grenzwert= | ||
Version vom 2. Januar 2012, 14:55 Uhr
Monotonie
Dieser Begriff des Ansteigens eines Funktionsgraphen fassen wir genauer und benennen ihn.
|
Eine Funktion |
Auch diesen Begriff des Fallens eines Funktionsgraphen fassen wir - analog zu oben - genauer und benennen ihn.
|
Eine Funktion |
Man könnte diese Begriffe monoton zunehmend und monoton abnehmend auch für die Funktionsgraphen übernehmen, hier verwendet man allerdings monoton steigend und monoton fallend.
|
Eine Funktionsgraph Eine Funktionsgraph |
|
Teste dich! Klicke im folgenden Quiz auf die richtigen Zuordnungen! |
 (streng monoton steigend) (!streng monoton fallend) (!weder noch)
(streng monoton steigend) (!streng monoton fallend) (!weder noch)
 (!streng monoton steigend) (streng monoton fallend) (!weder noch)
(!streng monoton steigend) (streng monoton fallend) (!weder noch)
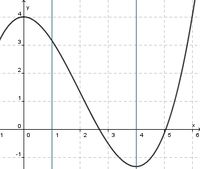 (!streng monoton steigend) (streng monoton fallend) (!weder noch)
(!streng monoton steigend) (streng monoton fallend) (!weder noch)
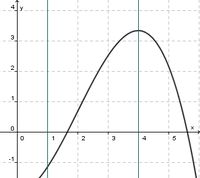 (streng monoton steigend) (!streng monoton fallend) (!weder noch)
(streng monoton steigend) (!streng monoton fallend) (!weder noch)
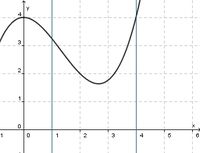 (!streng monoton steigend) (!streng monoton fallend) (weder noch)
(!streng monoton steigend) (!streng monoton fallend) (weder noch)
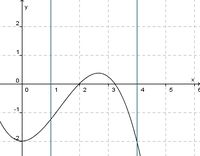 (!streng monoton steigend) (!streng monoton fallend) (weder noch)
(!streng monoton steigend) (!streng monoton fallend) (weder noch)
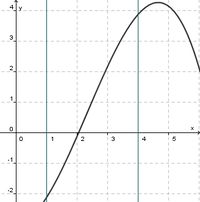 (streng monoton steigend) (!streng monoton fallend) (!weder noch)
(streng monoton steigend) (!streng monoton fallend) (!weder noch)
 in
in 
 in [0;1]
in [0;1] 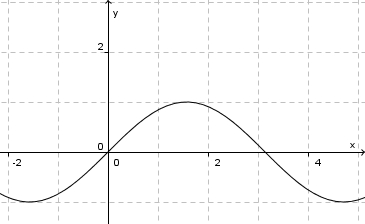
 in [0;3]
in [0;3] 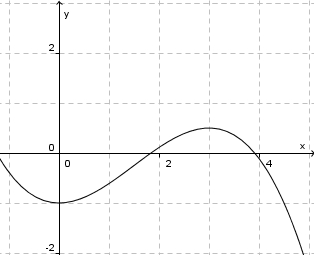
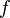 heißt streng monoton zunehmend im Intervall [a;b], wenn für alle
heißt streng monoton zunehmend im Intervall [a;b], wenn für alle ![x_1,x_2 \in [a;b]](/images/math/4/8/9/489a4ad3c2410297428c10a130e7cc95.png) gilt:
gilt: 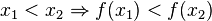


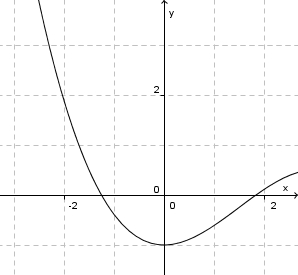
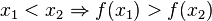
 heißt streng monoton steigend im Intervall [a;b], wenn die Funktion
heißt streng monoton steigend im Intervall [a;b], wenn die Funktion 
