Rationale Funktionen Polstellen: Unterschied zwischen den Versionen
| Zeile 22: | Zeile 22: | ||
Ist n ungerade, dann hat die Funktion <math>f:x\rightarrow \frac{1}{(x-x_0)^n}</math> mit <math>D = R\backslash \{x_0\}</math> an der Stelle <math>x = x_0</math> einen '''Pol mit Vorzeichenwechsel'''. | Ist n ungerade, dann hat die Funktion <math>f:x\rightarrow \frac{1}{(x-x_0)^n}</math> mit <math>D = R\backslash \{x_0\}</math> an der Stelle <math>x = x_0</math> einen '''Pol mit Vorzeichenwechsel'''. | ||
| + | }} | ||
| + | |||
| + | |||
| + | {{Aufgabe| | ||
| + | Ermittle bei den gegebenen Funktionen jeweils die Polstelle(n) der Funktion und beschreibe das Vorzeichenverhalten der Funktion bei Annäherung an die Polstelle(n). | ||
| + | |||
| + | a) <math>f</math> mit <math> f(x) = \frac{1}{x-2}</math> | ||
| + | |||
| + | b) <math>g</math> mit <math> g(x) = \frac{1}{2-x}</math> | ||
| + | |||
| + | c) <math>h</math> mit <math> h(x) = \frac{1}{(x-2)^2}</math> | ||
| + | |||
| + | d) <math>k</math> mit <math> k(x) = \frac{1}{(x-3)^7}</math> | ||
| + | |||
| + | e) <math>l</math> mit <math> l(x) = \frac{1}{(x-3)(x+2)}</math> | ||
| + | }} | ||
| + | |||
| + | |||
| + | {{Lösung versteckt|1= | ||
| + | |||
| + | a) x = 2; Pol 1. Ordnung; Pol mit Vorzeichenwechsel; Annäherung von links (x<2): <math>f(x) \rightarrow -\infty</math>; Annäherung von rechts (x>2): <math> f(x) \rightarrow \infty</math> | ||
| + | |||
| + | b) x = 2; Pol 1. Ordnung; Pol mit Vorzeichenwechsel; Annäherung von links (x<2): <math>g(x) \rightarrow \infty</math>; Annäherung von rechts (x>2): <math> g(x) \rightarrow -\infty</math> | ||
| + | |||
| + | c) x = 2; Pol 2. Ordnung; Pol ohne Vorzeichenwechsel; Annäherung von links (x<2): <math>h(x) \rightarrow \infty</math>; Annäherung von rechts (x>2): <math> h(x) \rightarrow \infty</math> | ||
| + | |||
| + | d) x = 3; Pol 7. Ordnung; Pol mit Vorzeichenwechsel; Annäherung von links (x<3): <math>k(x) \rightarrow -\infty</math>; Annäherung von rechts (x>3): <math> k(x) \rightarrow -\infty</math> | ||
| + | |||
| + | e) x = -2; Pol 1. Ordnung; Pol mit Vorzeichenwechsel; Annäherung von links (x<-2): <math>l(x) \rightarrow \infty</math>; Annäherung von rechts (x>-2): <math> f(x) \rightarrow -\infty</math><br> | ||
| + | x = 3; Pol 1. Ordnung; Pol mit Vorzeichenwechsel; Annäherung von links (x<3): <math>l(x) \rightarrow -\infty</math>; Annäherung von rechts (x>3): <math> f(x) \rightarrow \infty</math> | ||
| + | |||
| + | |||
| + | |||
}} | }} | ||
Version vom 4. April 2013, 11:21 Uhr
Die Funktion 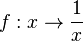 ist für
ist für 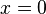 nicht definiert. Wie verhält sie sich in der Umgebung von
nicht definiert. Wie verhält sie sich in der Umgebung von 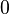 ? Je kleiner
? Je kleiner 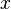 betragsmäßig wird, desto größer wird der Betrag von
betragsmäßig wird, desto größer wird der Betrag von  . Zeigt eine Funktion für einen x-Wert ein solches Verhalten, dann ist der x-Wert eine Definitionslücke und man bezeichnet diese Stelle als Polstelle.
. Zeigt eine Funktion für einen x-Wert ein solches Verhalten, dann ist der x-Wert eine Definitionslücke und man bezeichnet diese Stelle als Polstelle.
|
Ist an einer Definitionslücke
dann ist die Definitionslücke |
Beispiele:
1. Die Funktion 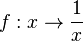 hat für
hat für 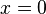 einen Pol 1. Ordnung (
einen Pol 1. Ordnung (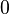 ist einfache Nullstelle des Nenners).
ist einfache Nullstelle des Nenners).
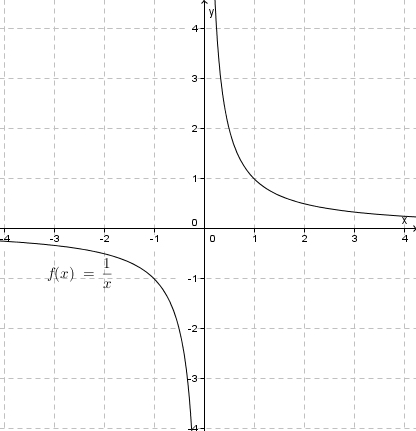
Nähert man sich von links an, also 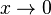 mit
mit 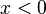 , dann streben die Funktionswerte nach
, dann streben die Funktionswerte nach 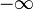 ; nähert man sich von rechts an, also
; nähert man sich von rechts an, also 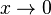 mit
mit 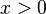 , dann streben die Funktionswerte nach
, dann streben die Funktionswerte nach  .
. 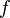 hat an
hat an 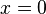 eine Polstelle mit Vorzeichenwechsel. Die Gerade
eine Polstelle mit Vorzeichenwechsel. Die Gerade 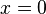 ist senkrechte Asymptote des Graphen von
ist senkrechte Asymptote des Graphen von 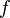 .
.
2. Die Funktion 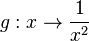 hat für
hat für 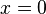 einen Pol 2. Ordnung (
einen Pol 2. Ordnung (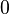 ist zweifache Nullstelle des Nenners).
ist zweifache Nullstelle des Nenners).
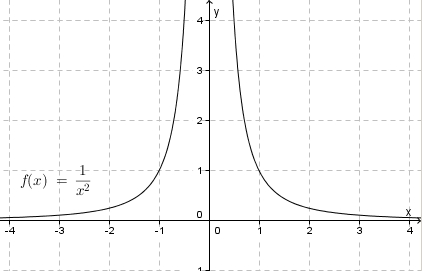
Nähert man sich von links oder von rechts an, also 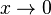 mit
mit 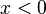 oder
oder 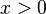 , dann streben die Funktionswerte in beiden Fällen nach
, dann streben die Funktionswerte in beiden Fällen nach  .
. 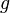 hat an
hat an 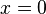 eine Polstelle ohne Vorzeichenwechsel. Die Gerade
eine Polstelle ohne Vorzeichenwechsel. Die Gerade 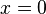 ist senkrechte Asymptote des Graphen von
ist senkrechte Asymptote des Graphen von 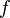 .
.
|
Man kann allgemein für eine gebrochen-rationale Funktion Ist n gerade, dann hat die Funktion Ist n ungerade, dann hat die Funktion |
|
Ermittle bei den gegebenen Funktionen jeweils die Polstelle(n) der Funktion und beschreibe das Vorzeichenverhalten der Funktion bei Annäherung an die Polstelle(n). a) b) c) d) e) |
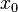 einer gebrochen-rationalen Funktion
einer gebrochen-rationalen Funktion 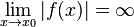 ,
,
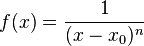 formulieren:
formulieren:
 mit
mit 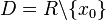 an der Stelle
an der Stelle 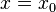 einen Pol ohne Vorzeichenwechsel.
einen Pol ohne Vorzeichenwechsel.
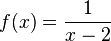

 mit
mit 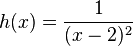
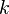 mit
mit 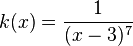
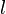 mit
mit 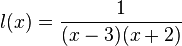
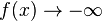 ; Annäherung von rechts (x>2):
; Annäherung von rechts (x>2): 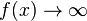
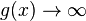 ; Annäherung von rechts (x>2):
; Annäherung von rechts (x>2): 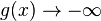
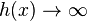 ; Annäherung von rechts (x>2):
; Annäherung von rechts (x>2): 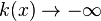 ; Annäherung von rechts (x>3):
; Annäherung von rechts (x>3): 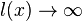 ; Annäherung von rechts (x>-2):
; Annäherung von rechts (x>-2): 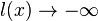 ; Annäherung von rechts (x>3):
; Annäherung von rechts (x>3): 
