|
|
| Zeile 1: |
Zeile 1: |
| | Du hast nun Funktionen als Objekte in der Mathematik kennengelernt. Wir wollen als Nächstes untersuchen, welche Eigenschaften Funktionen haben. | | Du hast nun Funktionen als Objekte in der Mathematik kennengelernt. Wir wollen als Nächstes untersuchen, welche Eigenschaften Funktionen haben. |
| | | | |
| − | =Monotonie=
| + | Als Eigenschaften wollen wir Monotonie, Grenzwert und Symmetrie betrachten. |
| | | | |
| − | {{Arbeiten|NUMMER=1|
| + | [[Funktionen_Einstieg/Monotonie]] |
| − | ARBEIT=
| + | |
| − | Betrachte die folgenden Funktionen im angegebenen Intervall. Die Funktionen sind durch Funktionsterm und Graph gegeben. <br>
| + | |
| | | | |
| − | a) <math>f:x \rightarrow x^2</math> in <math>R^+</math> <center>[[datei:Monotonie_quadratfunktion.jpg]]</center>
| + | [[Funktionen_Einstieg/Grenzwert]] |
| | | | |
| − | b) <math>f:x \rightarrow sin(x)</math> in [0;1] <center>[[datei:Montonie_sinusfunktion.jpg]]</center>
| + | [[Funktionen_Einstieg/Symmetrie]] |
| | | | |
| − | c) <math>f:x \rightarrow -\frac{1}{9}x^3 + \frac{1}{2}x^2-1</math> in [0;3] <center>[[datei:Monotonie_kubikfunktion.jpg]]</center
| |
| − |
| |
| − | Was fällt dir auf?
| |
| − | Was haben die drei Funktionsgraphen in den angegebenen Intervallen gemeinsam?
| |
| − | }}
| |
| − |
| |
| − | {{Lösung versteckt|
| |
| − | Alle drei Funktionsgraphen "steigen" in dem angegebenen Intervall an.}}
| |
| − |
| |
| − | Dieser Begriff des Ansteigens eines Funktionsgraphen fassen wir genauer und benennen ihn.
| |
| − |
| |
| − | {{Merke|
| |
| − | Eine Funktion <math> f</math> heißt '''streng monoton zunehmend''' im Intervall [a;b], wenn für alle <math> x_1,x_2 \in [a;b]</math> gilt: <math>x_1 < x_2 \Rightarrow f(x_1) < f(x_2)</math>
| |
| − | }}
| |
| − |
| |
| − |
| |
| − |
| |
| − | {{Arbeiten|NUMMER=2|
| |
| − | ARBEIT=
| |
| − |
| |
| − | Betrachte die folgenden Funktionen in den angegebenen Intervallen Quadratfunktion in den angegebenen Intervallen
| |
| − | </center>
| |
| − | a) <math>f:x \rightarrow x^2</math> in <math>R^-</math> <center>[[datei:Monotonie_quadratfunktion2.jpg]]</center><br>
| |
| − |
| |
| − | b) <math>f:x \rightarrow sin(x)</math> in [2;3] <center>[[datei:Montonie_sinusfunktion.jpg]]</center>
| |
| − |
| |
| − | c) <math>f:x \rightarrow -\frac{1}{9}x^3 + \frac{1}{2}x^2-1</math> in [-3;0] <center>[[datei:Monotonie_kubikfunktion2.jpg]]</center>
| |
| − |
| |
| − | Was stellst du nun fest? Was haben alle drei Graphen in den angegebenen Intervallen gemeinsam?
| |
| − | }}
| |
| − |
| |
| − | {{Lösung versteckt|
| |
| − | Alle drei Funktionsgraphen "fallen" in dem angegebenen Intervall.}}
| |
| − |
| |
| − |
| |
| − |
| |
| − | Auch diesen Begriff des Fallens eines Funktionsgraphen fassen wir - analog zu oben - genauer und benennen ihn.
| |
| − |
| |
| − | {{Merke|
| |
| − | Eine Funktion <math> f</math> heißt '''streng monoton abnehmend''' im Intervall [a;b], wenn für alle <math> x_1,x_2 \in [a;b]</math> gilt: <math>x_1 < x_2 \Rightarrow f(x_1) > f(x_2)</math>
| |
| − | }}
| |
| − |
| |
| − |
| |
| − |
| |
| − | Man könnte diese Begriffe '''monoton zunehmend''' und '''monoton abnehmend''' auch für die Funktionsgraphen übernehmen, hier verwendet man allerdings '''monoton steigend''' und '''monoton fallend'''.
| |
| − |
| |
| − | {{Merke|
| |
| − | Eine Funktionsgraph <math> G_f</math> heißt '''streng monoton steigend''' im Intervall [a;b], wenn die Funktion <math>f</math> dort streng monoton zunehmend ist, <br>
| |
| − | d.h.für alle <math> x_1,x_2 \in [a;b]</math> gilt: <math>x_1 < x_2 \Rightarrow f(x_1) < f(x_2)</math>
| |
| − |
| |
| − | Eine Funktionsgraph <math> G_f</math> heißt '''streng monoton fallend''' im Intervall [a;b], wenn die Funktion <math>f</math> dort streng monoton abnehmend ist, <br>
| |
| − | d.h. für alle <math> x_1,x_2 \in [a;b]</math> gilt: <math>x_1 < x_2 \Rightarrow f(x_1) > f(x_2)</math>
| |
| − | }}
| |
| − |
| |
| − | {{Arbeiten|NUMMER=3|ARBEIT=
| |
| − |
| |
| − | Teste dich! Klicke im folgenden Quiz auf die richtigen Zuordnungen!
| |
| − | }}
| |
| − |
| |
| − | <div class="multiplechoice-quiz">
| |
| − |
| |
| − | [[Bild:Monotonie_f1.jpg|200px]]
| |
| − | (streng monoton steigend) (!streng monoton fallend) (!weder noch)
| |
| − |
| |
| − | [[Bild:Monotonie_f2.jpg|200px]]
| |
| − | (!streng monoton steigend) (streng monoton fallend) (!weder noch)
| |
| − |
| |
| − | [[Bild:Monotonie_f5.jpg|200px]]
| |
| − | (!streng monoton steigend) (!streng monoton fallend) (weder noch)
| |
| − |
| |
| − | [[Bild:Monotonie_f3.jpg|200px]]
| |
| − | (!streng monoton steigend) (streng monoton fallend) (!weder noch)
| |
| − |
| |
| − | [[Bild:Monotonie_f6.jpg|200px]]
| |
| − | (streng monoton steigend) (!streng monoton fallend) (!weder noch)
| |
| − |
| |
| − | [[Bild:Monotonie_f4.jpg|200px]]
| |
| − | (!streng monoton steigend) (!streng monoton fallend) (weder noch)
| |
| − |
| |
| − | [[Bild:Monotonie_f7.jpg|200px]]
| |
| − | (streng monoton steigend) (!streng monoton fallend) (!weder noch)
| |
| − |
| |
| − | </div>
| |
| − |
| |
| − | Für die folgende Multiple-Choice-Aufgabe kannst du als Hilfe GeoGebra öffnen, dir die Graphen der Funktionen zeichnen lassen und dann die Fragen beantworten.
| |
| − |
| |
| − | <div class="multiplechoice-quiz">
| |
| − | <math>f:x \rightarrow x^2</math> im Intervall [2;8]
| |
| − | (streng monoton zunehmend) (!streng monoton abnehmend) (!weder noch)
| |
| − |
| |
| − | <math>f:x \rightarrow 2sin(x) + 3 </math> im Intervall [<math>\pi;\frac{3}{2}\pi</math>]
| |
| − | (!streng monoton zunehmend) (streng monoton abnehmend) (!weder noch)
| |
| − |
| |
| − | <math>f:x \rightarrow 2^x </math> im Intervall [-1;4]
| |
| − | (streng monoton zunehmend) (!streng monoton abnehmend) (!weder noch)
| |
| − |
| |
| − | <math>f:x \rightarrow log_2(x) </math> im Intervall [-1;4]
| |
| − | (streng monoton zunehmend) (!streng monoton abnehmend) (!weder noch)
| |
| − |
| |
| − | <math>f:x \rightarrow 4-x^2 </math> im Intervall [-1;4]
| |
| − | (!streng monoton zunehmend) (!streng monoton abnehmend) (weder noch)
| |
| − |
| |
| − | <math>f:x \rightarrow x^2+2x+1</math> im Intervall [2;8]
| |
| − | (streng monoton zunehmend) (!streng monoton abnehmend) (!weder noch)
| |
| − |
| |
| − | <math>f:x \rightarrow x^n</math> mit <math> n gerade</math> im Intervall [-4;-1]
| |
| − | (!streng monoton zunehmend) (streng monoton abnehmend) (!weder noch)
| |
| − |
| |
| − | <math>f:x \rightarrow x^n</math> mit <math> n ungerade</math> im Intervall [-3;9]
| |
| − | (streng monoton zunehmend) (!streng monoton abnehmend) (!weder noch)
| |
| − |
| |
| − | </div>
| |
| | | | |
| | =Grenzwert= | | =Grenzwert= |
Merke
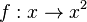
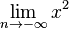
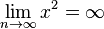und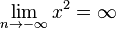 
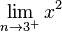heißt: x ist bei der Annäherung größer als 3, also z.B. 3,1 ; 3,01 ; 3,001 usw.
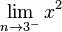heißt: x ist bei der Annäherung kleiner als 3, also z.B. 2,9 ; 2,99 ; 2,999 usw.
bzw.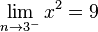 
.
